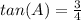Answer:
Option C.
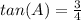
Explanation:
we know that
The tangent of angle A is equal to divide the opposite side to angle A (side BC) by the adjacent side to angle A ( side AB)
so
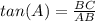
substitute the values
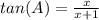
Applying the Pythagoras Theorem find the value of x
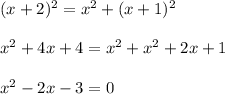
using a graphing calculator-----> solve the quadratic equation
The solution is x=3 -----> see the attached figure
substitute the value of x in the tan(A)