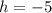Question

What is the value of k

Answer 1

Answer: k = -3

Explanation:

If we have a function f(x) defined in the real numbers we can transform it by moving it vertically doing
`y = f (x) + k`

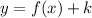
If k is greater than zero the graph of f(x) will move k units up. If k is less than zero the graph of f(x) will move k units down.

In the same way if you make the transformation
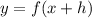`y = f (x + h)` then the graph of f(x) will move h units to the right if
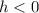`h <0` and move h units to the left if
`h> 0`.

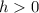In this problem we have to:

`f(x) =((1)/(3))^x`

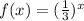
then we see that the graph of g(x) is displaced 3 units down. This means that
`k = -3`

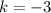
Also the graph of g(x) is equal to the graph of f(x) displaced 5 units to the right so that
`h = -5`