Question

At what point is the following function continuous?

Answer 1

Check the picture below.

something worth noticing
`\bf \cfrac{x^2-x-6}{x-3}\implies \cfrac{(\underline{x-3})(x+2)}{\underline{x-3}}\implies x+2`

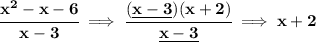
so, we're really graphing x+2, with a hole at x = 3, however, when x = 3, we know that f(x) = 5, but but but, when x = 3, x+2 = 5, so we end up with a continuous line all the way, x ∈ ℝ, because the "hole" from the first subfunction, gets closed off by the second subfunction in the piece-wise.