Question

PLEASE HELP ASAP!!! CORRECT ANSWER ONLY PLEASE!!!

Use technology or a z-score table to answer the question.

Lengths of newborn girls are normally distributed with a mean of 49.2 cm and a standard deviation of 1.8 cm. Consider a group of 2000 newborn girls.

Approximately how many girls will be 51 cm or shorter?

Answer 1

1683

Explanation:

1683

Answer 2

Answer: a) 1683

Explanation:

First, let's find the z-score using the formula:
`z=(x-\mu)/(\sigma)`

`z=(51-49.2)/(1.8)=(1.8)/(1.8)=\boxed{1.00}`

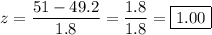
Next, refer to the z-score table below to find the value of 1.00

Look on the left side for 1.0 and the top for 0.00 to find the percent for 1.00. The table shows the percent above the MEAN so you need to add the percent below the mean (50%) to the value in the table.

0.3413

+ 0.5

0.8413

Now multiply that percent by the total number (2000) of girls to find out the quantity of girls who are 51 cm or shorter.

0.8413

× 2000

1682.6