Question

Helium is used for balloons because it is less dense than air. This is what allows helium balloons to rise. Suppose a helium- air mixture in a spherical balloon with a diameter of 1 foot has a density of 0.04 pounds per cubic foot. What is the weight of the gas mixture in the balloon?

A. About 0.02 pounds
B. About 0.03 pounds
C. About 0.04 pounds
D. About 0.52 pounds

Answer 1

Answer: Option A

About 0.02 pounds

Explanation:

We know that density is defined as:

`d = (m)/(v)`

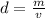
Where

d is the density

m is the mass

v is the volume

We want to calculate the helium-air mass of a spherical balloon and we know its density and the diameter of the balloon.

Then we can calculate its mass.

1) We use the diameter of the spherical globe to calculate its volume.

The volume of a sphere is:

`V = (4)/(3)\pi r ^ 3`

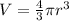
and

`radius = (diameter)/(2)`

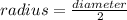
So

`V = (4)/(3)\pi((d)/(2)) ^ 3\\\\V = (4)/(3)\pi((1)/(2)) ^ 3`

`V= 0.5236\ ft^3`

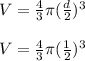
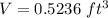
2) Use the density formula to find the mass

`d = (m)/(v)`

`m = vd`

`m = (0.5236\ ft^3)*(0.04\ (pound)/(ft^3))\\\\m = 0.02\ pounds`

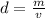
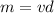
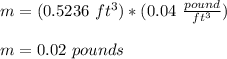
The answer is 0.02 pounds