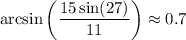Question

PLEADE HELP! find the value of X. Round to the nearest tenth.

Answer 1

x ≈ 28.2

Explanation:

Using the sine rule then

`(sinx)/(15)` =
`(sin27)/(11)` ( cross- multiply )

11sinx = 15sin27° ( divide both sides by 11 )

sin x =
`(15sin27)/(11)` ≈ 0.61907..

x =
`sin^(-1)`(0.61907 ) ≈ 28.2

Answer 2

As suggested, we have to use the law of sines: it states that the ratio between a side and the sine of the opposite angle is constant in every triangle.

We know that one side length is 11, and the opposite angle is 27°

Another side length is 15, and the opposite angle is x.

So, the law of sines states that

`(11)/(\sin(27))=(15)/(\sin(x))`

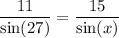
Solving for
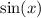`\sin(x)` we have

`\sin(x) = (15\sin(27))/(11)`

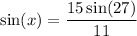
Which implies

`x=\arcsin\left((15\sin(27))/(11)\right)`

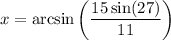
Put this into a calculator to get

`\arcsin\left((15\sin(27))/(11)\right)\approx 0.7`