Answer:
x2+y2−10x−12y+60=0
Explanation:
The standard form of the equation of a circle is usually given in the form;
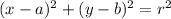
The center of the circle is (a, b) while r denotes the radius.
The general form of the equation of a circle is obtained by expanding the brackets, collecting like terms and simplifying.
The standard form in this case has been given as;
(x−5)^2+(y−6)^2=1
We simply open the brackets and simplify to obtain the general form;
(x−5)^2 = (x−5) (x−5)
= x^2 -5x -5x +25
= x^2 -10x +25
(y−6)^2 = (y-6) (y-6)
= y^2 -6y - 6y +36
= y^2 -12y +36
(x−5)^2+(y−6)^2 = x^2 -10x +25 + y^2 -12y +36 = 1
x^2 -10x +25 + y^2 -12y +36 = 1
x^2 + y^2 -10x -12y +25 + 36 = 1
x^2 + y^2 -10x -12y + 60 = 0