Answer:
C) Acceleration 1 - constant acceleration. Acceleration 2 - varied acceleration.
Step-by-step explanation:
In a velocity-time graph, the acceleration corresponds to the slope of the curve.
In fact, acceleration is defined as the ratio between the change in velocity and the time interval:

However, we see that in a velocity-time graph,
 corresponds to the increment in the y-variable (
corresponds to the increment in the y-variable (
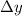 ), while
), while
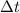 corresponds to the increment in the x-variable (
corresponds to the increment in the x-variable (
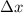 ). Therefore, acceleration can also be written as
). Therefore, acceleration can also be written as
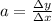
which is exactly the definition of slope of the curve.
Now we notice that:
- For object 1, the slope is constant: this means that the acceleration is constant
- For object 2, the slope varies: this means that the acceleration varies as well