Question

Given that a3 = 12 and a5 = 48, find the explicit rule for the geometric sequence, given that the common ratio is positive

Answer 1

hello : q=2

Explanation:

look this solution :

Answer 2

see explanation

Explanation:

The n th term of a geometric sequence is

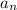`a_(n)` = a
`r^(n-1)`

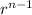
where a is the first term and r the common ratio, thus

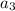`a_(3)` = ar² = 12 → (1)

`a_(5)` = a
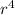`r^(4)` = 48 → (2)

Divide (2) by (1)

`(ar^4)/(ar^2)` =
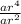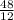`(48)/(12)` = 4

r² = 4 ⇒ r = ± 2 ⇒ r = 2 ← since r > 0

Substitute r = 2 into (1) for corresponding value of a

4a = 12 ⇒ a = 3

Hence

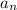`a_(n)` = 3
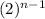`(2)^(n-1)` ← explicit rule