Question

What is the area of this triangle? Enter your answer as a decimal in the box. Round only your final answer to the nearest hundredth. ft²

one side of the triangle is 3.8 ft and the opposite side is 4.3 ft. the angle connecting the two sides is 53 degrees.

*edit: i just took the test and the answer is 6.52

Answer 1

Yes you are correct the answer is 6.52

Answer 2

The area of the given triangle is 6.52 square feet.

Explanation:

It is given that one side of the triangle is 3.8 ft and the opposite side is 4.3 ft. the angle connecting the two sides is 53 degrees.

Thus, Using the formula for the area of triangle, we get

`{\text{Area of triangle}}=(absinC)/(2)`

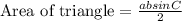
Now, substituting the given values, we get

`A=((3.8)(4.3)sin53^(\circ))/(2)`

⇒
`A=((16.34)(0.799))/(2)`

⇒
`A=(13.05)/(2)`

⇒
`A=6.52 ft^2`

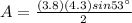
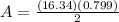
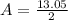
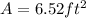
Therefore, the area of the given triangle is 6.52 square feet.