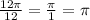Answer:
Circumference of the circle:
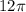
Ratio of the circumference to the diameter:

Explanation:
First, we are finding the circumference of the circle using the circumference formula:
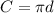
were
 is the circumference of the circle
is the circumference of the circle
 is the diameter of the circle
is the diameter of the circle
We know from the problem that the diameter of our circle is 12cm, so
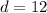 .
.
Replacing values:
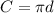
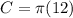

Now, to find the ratio of the circumference to the diameter, we just need to divide the circumference of our circle by the diameter:
ratio =