Question

Need answer please!!!!

Answer 1

B

Explanation:

Using the rules of radicals/ exponents

•
`(√(a) )/(√(b) )` ⇔
`\sqrt{(a)/(b) }`, hence

`\sqrt{(c^2d^6)/(4c^3d^-4) }` ← simplify

=
`\sqrt{(1)/(4)((1)/(c))(d^(10))  }`

=
`(d^5)/(2√(c) )` → B

Answer 2

For this case we must simplify the following expression:

`\frac {\sqrt {c ^ 2 * d ^ 6}} {\sqrt {4c ^ 3 * d ^ {- 4}}}`

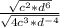
By definition of properties of powers and roots, we have to:

`\sqrt [n] {a ^ m} = a ^ {\frac {m} {n}}`

Then, rewriting the expression:

`\frac {c ^ {\frac {2} {2}} * d {\frac {6} {2}}} {2 * c ^ {\frac {2} {2}} * d ^ {\frac { -4} {2}} * \sqrt {c}}`

`(c*d^3)/(2c*d^(-2)*√(c))`

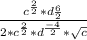
By definition of properties of division of powers of equal base we have to;

`\frac {a ^ m} {a ^ n} = a ^ {m-n}`

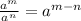
Rewriting the expression:

`(c*d^(3-(-2)))/(2c*√(c))\\(c*d^5)/(2c*√(c))\\(d^5)/(2√(c))`

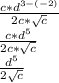
Answer:

Option B