Question

For a group experiment, your science class measured the fine-particulate concentrations in the air at random places around campus, and estimated a sample average of 22 μg/m3 (micrograms per cubic meter). If 144 readings were taken, and the standard deviation of the sample measurements was 4 μg/m3, you are 99.7% confident that actual concentration of fine particulates at the school is

Answer 1

21 ug/m^3 - 23 ug/m^3

Explanation:

Just got it right

Answer 2

The class interval is from (22.8748 ug/m³ - 21.1252 ug/m³).

Explanation:

Given : Sample size(n)= 144

Sample mean
`\mu= 22 ug/m^3`

Standard deviation
`\sigma=3.5 ug/m^3`

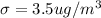
To find : 99.7% confident that actual concentration of fine particulates at the school is?

Solution :

The formula for confidence interval is

`CI=\mu \pm z*(\sigma)/(√(n))`

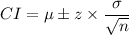
Substituting the values in the formula,

`CI=22 \pm 3*(3.5)/(√(144))`

`CI=22 \pm 3*(3.5)/(12)`

`CI=22 \pm 3* 0.2916`

`CI=22 \pm 0.8748`

`CI=22+0.8748, 22-0.8748`

`CI=22.8748, 21.1252`

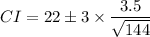
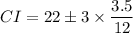
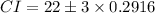
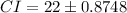
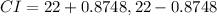
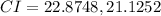
Therefore, The class interval is from (22.8748 ug/m³ - 21.1252 ug/m³).