Answer:
0
3
Explanation:
The given functions are
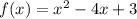
We can rewrite this function in the vertex form to obtain;
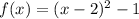
This is the graph of the parent function
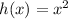 shifted, 2 units to the right and 1 unit down.
shifted, 2 units to the right and 1 unit down.
The second function is

This is the graph of the parent function
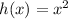 reflected in the x-axis and shifted up 3 units.
reflected in the x-axis and shifted up 3 units.
The graph of the two functions are shown in the attachment.
The solution to f(x)=g(x) is where the two graphs meet.
The two graphs intersected at
(0,3) and (2,-1)