Question

This is calculus

using L'hospitals rule
Lim x --> 0 (1+2x)^(-3/x)

Answer 1

De l'Hospital rule applies to undetermined forms like

`(0)/(0),\quad(\infty)/(\infty)`

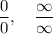
If we evaluate your limit directly, we have

`\displaystyle \lim_(x\to 0)(1+2x)^{-(3)/(x)} = 1^\infty`

which is neither of the two forms covered by the theorem.

So, in order to apply it, we need to write the limit as follows: we start with

`f(x)=(1+2x)^{-(3)/(x)}`

Using the identity
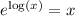`e^(\log(x))=x`, we can rewrite the function as

`f(x)=e^{\log\left((1+2x)^{-(3)/(x)}\right)}`

Using the rule
`\log(a^b)=b\log(a)`, we have

`f(x)=e^{-(3)/(x)\log(1+2x)}`

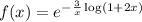
Since the exponential function
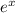`e^x` is continuous, we have

`\displaystyle \lim_(x\to 0) e^(f(x)) = e^{\lim_(x\to 0) f(x)}`

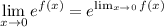
In other words, we can focus on the exponent alone to solve the limit. So, we're focusing on

`\displaystyle \lim_(x\to 0) -(3)/(x)\log(1+2x)`

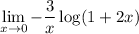
Which we can rewrite as

`\displaystyle \lim_(x\to 0) -(3)/(x)\log(1+2x) = -3\lim_(x\to 0)(\log(1+2x))/(x)`

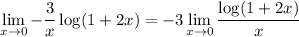
Now the limit comes in the form 0/0, so we can apply the theorem: we derive both numerator and denominator to get

`\displaystyle -3\lim_(x\to 0)(\log(1+2x))/(x) = -3 \lim_(x\to 0)((2)/(1+2x))/(1) = -3\cdot 2 = -6`

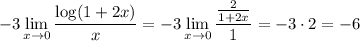
So, the limit of the exponent is -6, which implies that the whole expression tends to

`e^(-6)=(1)/(e^6)`