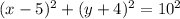Answer:
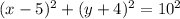
Explanation:
The standard of equation of a circumference has the form:

Where the point (a,b) is the center of the circumference and r is the radius.
You know the center of the circumference: (5,-4).
Substitute this point into the equation of the circumference:
a=5 and b=-4
Then:
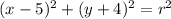
Now you need to find the radius. Substitute the point (-3,2) into the circumference and solve for r:
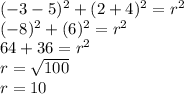
The equation of this circumference is: