Question

HELPPPPPPPPP i have the most trouble with math what’s the answer??

Answer 1

Second Option

`(5√(11) +5√(3))/(8)`

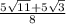
Explanation:

To simplify the expression you must multiply the numerator and the denominator of the fraction by the conjugate of the denominator.

If you have an expression of the form

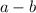`a -b` then its conjugate will be
`a + b.`

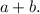
The result of that multiplication will be

`a ^ 2 -b ^ 2`

Therefore we have the expression

`(5)/(√(11) -√(3))`

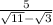
By multiplying by the conjugate of the denominator we have

`(5)/(√(11) -√(3))*(√(11) +√(3))/(√(11) +√(3))\\\\\\(5(√(11) +√(3)))/((√(11))^2 -(√(3))^2)\\\\\\(5√(11) +5√(3))/(11 -3)\\\\\\(5√(11) +5√(3))/(8)`