Question

Two clocks are circular. Clock A has twice the area Clock B. How many times greater is the radius of Clock A than the Clock B radius?

Answer 1

The radius of the circle A is
`√(2)` times greater than the radius of circle B

Explanation:

step 1

Find the scale factor

we know that

If two figures are similar, then the ratio of its areas is equal to the scale factor squared

Let

z-----> the scale factor

x----> the area of the clock A

y----> the area of the clock B

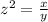`z^(2)=(x)/(y)` -----> equation A

we have

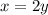`x=2y` -----> equation B

substitute equation B in equation A

`z^(2)=(2y)/(y)=2`

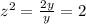
square root both sides

`z=√(2)`

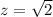
step 2

Find how many times greater is the radius of Clock A than the Clock B radius

we know that

If two figures are similar, then the ratio of its corresponding sides is equal to the scale factor

Let

z-----> the scale factor

x----> the radius of the clock A

y----> the radius of the clock B

`z=(x)/(y)`

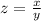
we have

`z=√(2)`

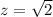
substitute

`√(2)=(x)/(y)`

`x=√(2)y`

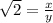
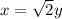
therefore

The radius of the circle A is
`√(2)` times greater than the radius of circle B