Question

Y=-x^2+6x-5

1. What is the vertex?
2. Does it open up or down?
3. What is the y-intercept?

Answer 1

1. Vertex: (3,4)

2. It opens down.

3. The y-intercept is -5

Explanation:

1. For a quadratic function in the form
`y=ax^2+bx+c`, you can calculate the x-coordinate of the vertex with:

`x=(-b)/(2a)`

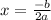
Then, given the function
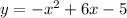`y=-x^2+6x-5`, you can identify that:

`b=6\\a=-1`

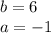
Substituting, you get:

`x=(-6)/(2(-1))=3`

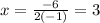
Substitute
`x=3` into
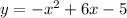`y=-x^2+6x-5` to find the y-coordinate of the vertex:

`y=-(3)^2+6(3)-5=4`

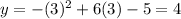
Then the vertex is at (3,4)

2. You identified above the value of "a". This is:

`a=-1`

Then, since
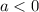`a<0`, the parabola opens down.

3. Substitute
`x=0` into the function and solve for "y":

`y=-(0)^2+6(0)-5\\y=-5`

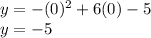
Therefore, the y-intercept is -5