Answer:
x = 1.91
Explanation:
* We have exponential equation to solve it lets study some rules
- If a^m = a^n ⇒ then m = n
- If a^m = b^m ⇒ then a = b or m = 0
- If a^m = b^n ⇒ log(a^m) = log(b^n) ⇒ m log(a) = n log(b)
* You can use log or ln
* Lets solve the problem
∵
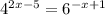 ⇒ insert log to both sides
⇒ insert log to both sides
∴

-

∴ (2x - 5)log(4) = (-x + 1)log(6) ⇒ open the brackets
∴ 2xlog(4) - 5log(4) = -xlog(6) + log(6) ⇒ collect x in one side
∴ 2xlog(4) + xlog(6) = log(6) + 5log(4) ⇒ take x as a common factor
∴ x(2log(4) + log(6)) = log(6) + 5log(4)
- divide both sides by coefficient of x

∴ x = 1.91