Question

What is the standard form of each circle? (questions 19&20)

Answer 1

`(x+18)^2 + (y+9)^2 = 1`

Explanation:

A circle is typically written in Vertex Form often called Standard Form by some. Its form is
`(x-h)^2 + (y-k)^2 = r^2` where (h,k) is the center and r is the radius. When given the end points of the diameter of the circle, find the distance between the points and halve it for the radius. To identity the vertex, find the midpoint of the diameter.

Radius

Use the distance formula to find the length of the segment by substituting in the end points.

`d = √((x_2-x_1)^2 + (y_2-y_1)^2) \\d = √((-17--19)^2 + (-9--9)^2) \\d = √((2)^2 + (0)^2) \\d = √(4 + 0) \\d = √(4) \\d=2`

The diameter is 2 and the radius is 1.

Vertex

To find the vertex, find the midpoint of the segment using the midpoint formula.

`((x_1+x_2)/(2), (y_1+y_2)/(2))\\\\((-17+-19)/(2), (-9 + -9)/(2))\\\\  (-18, -9)`

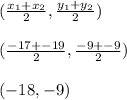
Write the equation of the circle as
`(x+18)^2 + (y+9)^2 = 1`