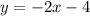Answer:
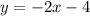
Explanation:
The slope-intercept form of the line is:

Where m is the slope and b the intersection with the y-axis.
You can see in the graph that line intersect the y-axis at
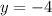 , then:
, then:
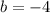
Choose two points and substitute them into the formula for calculate the slope. This is:

Let's pick the points (-4,4) and (-6,8). The the slope is:
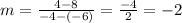
Substituting into the equation, you get: