ANSWER
She counted the squares instead of using the scale.
EXPLANATION
The slope of a line is calculated using the formula
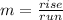
This is the same as:
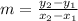
The given straight line passes through:
(4,24) and (16,18).
We plug in the values to obtain,

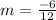
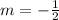
This is the correct expression for the slope.
But Willa mistakenly counted the squares instead of using the scale and had

Each box on the y-axis is 3 units and each box on the x-axis is 2 units.
The rise is one box along the y-axis which is equal to 3 units the run is 3 boxes which is equal to 6 units.
The line has a negative slope.
Because she just counted the boxes and did use the scale, she got a wrong answer.