Question

Find the x-intercept of a porabola with a vertex of (-3,-14) and y-intercept (0,-13)

Answer 1

see explanation

Explanation:

The equation of a parabola in vertex form is

y = a(x - h)² + k

where (h, k) are the coordinates of the vertex and a is a multiplier

here (h, k) = (- 3, - 14), thus

y = a(x + 3)² - 14

To find a substitute (0, - 13) into the equation

- 13 = 9a - 14 ( add 14 to both sides )

9a = 1 ( divide both sides by 9 )

a =
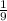`(1)/(9)`, hence

y =
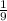`(1)/(9)`(x + 3)² - 14 ← equation of parabola

To find the x- intercepts let y = 0

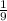`(1)/(9)`(x + 3)² - 14 = 0

Multiply through by 9

(x + 3)² - 126 = 0 ( add 126 to both sides )

(x + 3)² = 126 ( take the square root of both sides )

x + 3 = ±
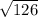`√(126)` = ± 3
`√(14)`

Subtract 3 from both sides

x = - 3 ± 3
`√(14)`

The x- intercepts are therefore

x = - 3 - 3
`√(14)` ≈ - 14.22 ( to 2 dec. places ) or

x = - 3 + 3
`√(14)` ≈ 8.22 ( to 2 dec. places )