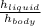Question

a uniform rod is hung at onen end and is partially submerged in water. If the density of the rod is 5/9 than of wter, find the fraction of the legth tof the rod above water

Answer 1

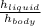`(h_(liquid) )/( h_(body) )` = 5/9

Step-by-step explanation:

This is an exercise that we can solve using Archimedes' principle which states that the thrust is equal to the weight of the desalted liquid.

B = ρ_liquid g V_liquid

let's write the translational equilibrium condition

B - W = 0

let's use the definition of density

ρ_body = m / V_body

m = ρ_body V_body

W = ρ_body V_body g

we substitute

ρ_liquid g V_liquid = ρ_body g V_body

`(\rho_(body) )/(\rho_(liquid) ) } = (V_(liquid) )/(V_(body) ) }`

In the problem they indicate that the ratio of densities is 5/9, we write the volume of the bar

V = A h_bogy

Thus

`(V_(liquid) )/(V_(1body) ) = ( h_(liquid) )/(h_(body) )`

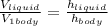
we substitute

5/9 =
`(h_(liquid) )/( h_(body) )`