Answer:
31.
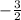
32. "for every unit increase in the number of shirts sold, there is an increase of 8 in profit"
33. (6,9)
34. (15, 40)
35. (8,8)
Explanation:
31.
The rate of change from this line would be the change in y over the change in x (or the slope). Basically we select 2 points at random on the line and see how many units we go in y direction and how many units we go in x direction to arrive at the second point.
Letting each unit as 1 unit in the coordinate grid, let's select the first point as (-2,2) and second point as (0,-1) [these are the 2 points given in the grid]
Now, we see that to go from (-2,2) to (0,-1), we need to go -3 units in y and +2 units in x. So the rate of change is change in y divided by change in x. Hence:
rate of change =
32.
If the function is given in the form f(x) = ax + b, the rate of change is the slope, which is a. This means for every unit of change in x, there is a unit change in y.
Looking at the function given, we see the rate of change is 8. Thus we can say that "for every unit increase in the number of shirts sold, there is an increase of 8 in profit"
33.
Dilation using a scale factor of 1/2 means changing coordinates of (x,y) into (1/2x,1/2y). This means making both the x coordinate and y coordinate, half of the original.
Hence (12,18) becomes (6,9).
34.
Dilating using a scale factor of 5 means changing coordinates of (x,y) into (5x,5y). It means multiply both the x and y coordinates by 5.
Hence, (3,8) would become (3*5, 8*5) = (15,40)
35.
Here, x coordinate is the first one, x = 5. The y coordinate is the second one, y = 10. Translation of right 3 places means "add 3 to the x coordinate". Also, translation down 2 units means "subtract 2 from y coordinate".
Hence, (5,10) would become (5+3, 10-2) = (8, 8)