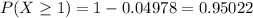Answer:
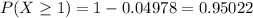
Explanation:
Step(i):-
Let 'X' be the random variable in Poisson distribution
Mean of the Poisson distribution λ or ∝ = 3 per (cm³)
Given n = 5 samples

Step(ii):-
The probability that at least one sample will contain one or more bacterial colonies
P(X≥1) = 1-P(X< 1)
= 1 - P( X=0)