Answer: Option B.
Explanation:
You neeed to use the trigonometric identity:
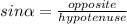
As you need to find sin∠A, you can identify in the right triangle that:
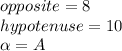
Finally, substitute the angle, the lenght of the opposite side and the lenght of the hypotenuse into
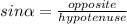 to find sin∠A. Then, you get:
to find sin∠A. Then, you get:
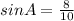
Reducing the fraction:
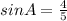
This is the option B.