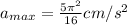Answer:
a)
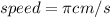
b)
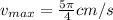
c)
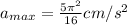
Step-by-step explanation:
The very first thing we must do in order to solve this problem is to find an equation for the simple harmonic motion of the given particle. Simple harmonic motion can be modeled with the following formula:
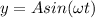
where:
A=amplitude
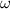 = angular frequency
= angular frequency
t=time
we know the amplitude is:
A=5.0cm
and the angular frequency can be found by using the following formula:
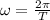
so our angular frequency is:

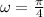
so now we can build our equation:
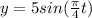
we need to find the speed of the particle when it is 3m from the centre of its motion, so we need to find the time t when this will happen. We can use the equation we just found to get this value:
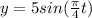
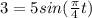
so we solve for t:

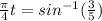
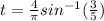
you can directly use this expression as the time or its decimal representation:
t=0.81933
since we need to find the speed of the particle at that time, we will need to get the derivative of the equation that represents the particle's position, so we get:
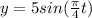

which simplifies to:
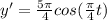
and we can now substitute the t-value we found previously, so we get:
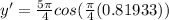
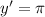
so its velocity at that point is
 cm/s
cm/s
b) In order to find the maximum velocity we just need to take a look at the velocity equation we just found:
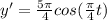
its amplitude will always give us the maximum velocity of the particle, so in this case the amplitude is:
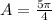
so:
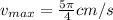
c) we can use a similar procedure to find the maximum acceleration of the particle, we just need to find the derivative of the velocity equation and determine its amplitude. So we get:

We can use the chain rule again to find this derivative so we get:

so when simplified we get:
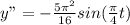
its amplitude is:

so its maximum acceleration is: