Question

What is the solution of (4x-16) ^ 1/2=36

Answer 1

x = 328

Explanation:

(4x-16) ^ 1/2=36

Raise both sides by the power of 2

((4x - 16)^1/2)^2 = 36^2

4x - 16 = 1296

4x = 1296 + 16

4x = 1312

x = 328

Answer 2

Hello!

The answer is:

The solution of the problem is 328

Why?

First, we need to remember the following roots property:

`\sqrt[n]{a^(m) }=a^{ (m)/(n)}`

Then, the expression:

`(4x-16)^{(1)/(2) }`

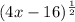
Can be written as:

`(4x-16)^{(1)/(2)}=\sqrt[2]{4x-16}`

Now, rewriting the equation, we have:

`\sqrt[2]{4x-16}=36`

Squaring both sides, and groping like terms, we have:

`(\sqrt[2]{4x-16})^(2) =36^(2)\\\\4x-16=1296\\4x=1296+16\\4x=1312\\x=(1312)/(4)=328`

Hence,

`x=328`

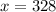
The solution of the problem is 328

Have a nice day!