Answer:
128.67 ft
Explanation:
Topic: Trigonometric Functions
You should be familiar with the concept of trigonometric functions because this topic involves degrees of traingles with the lenght of their sides, as you can see in the image, you need to remember which trigonometric function involves both sides (not hypotenuse) of a triangle, let's review
O= opposite, A = adjacent, H = Hypotenuse, α = 65°
sen (α) =

cos(α) =
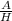
tan(α) =
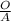
guess which of them works? yeah its tan(α), so we have that
tan(65°) =

findig h in the previous equation we have that
60 ft * tan(65°) = h
computing in a calculator it means that the height (h) of the tower is
h = 128. 67 ft