Answer:
The solution is: (1,-3)
Explanation:
Given equations are:
x-y=4 Eqn 1
4x+y = 1 Eqn 2
Substitution involves finding value of a variable from one equation and putting in other equation
From equation 1
x = y + 4
Putting in equation 2
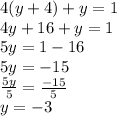
Putting y = -3 in equation 1
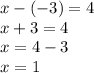
Hence,
The solution is: (1,-3)