ANSWER
745.31
Step-by-step explanation
The composite figure is made up of a rectangle and a semicircle.
The area of the rectangular portion
is
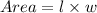
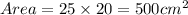
The semicircle has a radius of
r=12.5cm
The area of the semicircular portion is
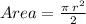
Substitute the value of r and π=3.14 to obtain,
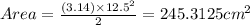
We add the two areas to obtain, the area of the composite figure.
500+245.3125=745.31 cm² to the nearest hundredth.