Question

A student hangs a weight on a newtonmeter. The energy currently stored in the spring in the newton meter is 0.045N. The student then increases the weight on the newtonmeter by 2N. If the spring constant is 400N/m what is the total extension of the spring?

Answer 1

Answer: 0.02m

Step-by-step explanation:

The other guy is completely wrong. He literally just used the energy store value to fill in the force for the Hooke's Law equation.

Firstly, we calculate the extension using this equation:

Elastic Potential Energy = 0.5 x Spring Constant x (Extension^2)

so 0.045 = 0.5 x 400 x e^2

so e =
`\sqrt[]{(0.045)/(0.5X400) }`

so e = 0.015

Now we just need to calculate the extension of 2N, then add it on to 0.015

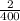`(2)/(400)` = 0.005

0.015 + 0.005 = 0.02

The answer is 0.2m

[Also the other guy cannot be correct because his final answer is equal to just the 2N weight, if you can understand what I mean]

Hope this helps. Thanks. Just posted this cuz didn't want people to get the wrong answer.

Answer 2

5x10^-3

Step-by-step explanation:

Hooke's Law states that the force needed to compress or extend a spring is directly proportional to the distance you stretch it.

Hooke's Law can be represented as

F = kx,

where F is the force

k is the spring constant

x is the extension of the material

Plug values in the equation

Step 1 find the original extension

0.045 = (400)x

x = 1.125x 10^-4 m d

Step 2 find the new extension

0.045+2 = 400(x)

2.045 = 400x

x = 5.1125x10^-3

Step 3 subtract the new extension with original

Total extension of the spring = 5.1125x10^-3 - 1.125x 10^-4 m = 5x10^-3