Answer:
The lines KL and MN perpendicular to each other.
Explanation:
If a line passes through two points, then the slope of the line is

From the given graph it is clear that coordinates of points on line KL are K(-8,2) and L(6,2).
The slope of line KL is
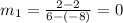
The slope of line KL is 0, it means it is a horizontal line.
From the given graph it is clear that coordinates of points on line MN are M(-4,8) and N(-4,-6).
The slope of line MN is
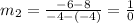
The slope of line MN is 1/0 or undefined, it means it is a vertical line.
We know that vertical and horizontal lines are perpendicular to each other. Therefore the lines KL and MN perpendicular to each other.