Question

Your grandmother wants to spend at least $40 but no more than $60 on school clothes for you. T-shirts sell for $10 and pants sell for $20. How many t-shirts and pants could she buy?

Write a system of two inequalities that describes the situation.
Write two possible solutions to the problem.

Answer 1

Part a) The system of two inequalities that describe the situation is

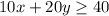`10x+20y\geq 40` and
`10x+20y\leq 60`

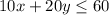
Part b) Two possible solutions are the points (2,2) and (2,1)

Explanation:

step 1

Find the system of inequalities

Let

x------> the number of t-shirts

y----> the number of pants

we know that

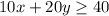`10x+20y\geq 40` -----> inequality A

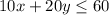`10x+20y\leq 60` ------> inequality B

using a graphing tool

The solution of the system of inequalities are all positive whole numbers in the shaded area

see the attached figure

step 2

Find two possible solutions

we know that

If a ordered pair is a solution of the system of inequalities, then the ordered pair must satisfy both inequalities

Two possible solutions are the points (2,2) and (2,1)

1) (2,2) -------> two t-shirts and two pants

substitute in both inequalities

`x=2,y=2`

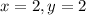
Inequality A

`10(2)+20(2)\geq 40`

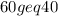`60 geq 40` ------> is true

Inequality B

`10(2)+20(2)\leq 60`

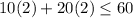
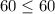`60\leq 60` ----> is true

therefore

the ordered pair (2,2) is a possible solution

2) (2,1) -------> two t-shirts and one pant

substitute in both inequalities

`x=2,y=1`

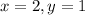
Inequality A

`10(2)+20(1)\geq 40`

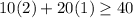
`40 geq 40` ------> is true

Inequality B

`10(2)+20(1)\leq 60`

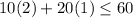
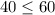`40\leq 60` ----> is true

therefore

the ordered pair (2,1) is a possible solution