Question

Two samples of the same ideal gas have the same pressure and density. Sample b has twice the volume of sample a. What is the rms speed of the molecules in sample b?

Answer 1

`√(2)v_(rms A)`

Step-by-step explanation:

First of all, let's remember that the kinetic energy K of a gas is directly proportional to its absolute temperature T:

`K\propto T`

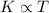
and the kinetic energy can be rewritten as

`K=(1)/(2)mv_(rms)^2`

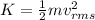
where m is the mass of the particles in the gas and
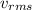`v_(rms)` is the rms speed. So, we have

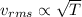`v_(rms)\propto √(T)` (1)

which means that the rms speed is proportional to the square of the temperature of the gas.

So we need to find how the temperature of the gas changes. This can be done by using the ideal gas law:

`T=(pV)/(nR)`

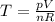
where p is the gas pressure, V the volume, n the number of moles and R the gas constant.

In this problem, we have:

Gas A and Gas B have same pressure:
`p_A = p_B`

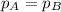
While sample B has twice the volume of sample A:

`V_B = 2 V_A`

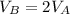
The two samples have same density, and since they are made of the same gas, the number of moles will also be the same:

`n_A = n_B`

So, the temperature of gas B will be

`T_B=(p_B V_B)/(nR)=(p_A (2 V_A))/(nR)=2 T_A`

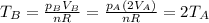
So, the temperature of gas B is twice that of gas A; as a result, the rms speed of the molecules in gas B will be

`v_(rms B)\propto √(2T_A)= √(2) √(T_A)= √(2)v_(rms A)`

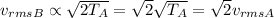
so it will be
`√(2)` times the rms speed of the molecules in gas A.