Question

The length of a rectangle is 3 centimeters less than four times its width. Its area is 10 square centimeters. Find the dimensions of the rectangle.

Answer 1

W = 2 cm

L = 5 cm

Explanation:

A rectangle is a four sided shape with 4 perpendicular angles. It has two pairs of parallel sides which are equal in distance: width and length. Its area, the amount of space inside it, can be found using the formula A = l*w. If the area is 10 cm² and the length is "3 cm less than 4 times the width" or 4w - 3, you can substitute and solve for w.

A = l*w

10 = (4w - 3)(w)

10 = 4w² - 3w

Subtract 10 from both sides to make the equation equal to 0. Then solve the quadratic by quadratic formula.

4w² - 3w - 10 = 0

Substitute a = 4, b = -3 and c = -10.

`w = (3 +/- √((-3)^2 - 4(4)(-10)) )/(2(4)) = (3 +/- √(9 +160)) )/(8) =  (3 +/- √(169) )/(8) = (3+/-13)/(8)`

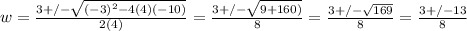
There are two possible solutions which can be found.

3 + 13 / 8 = 16/ 8 = 2

3 - 13 / 8 = -10/8 = -5/4

Since w is a side length or distance, it must be positive so w = 2 cm.

If the width is 2 cm then the length is 4(2) - 3 = 8 - 3 = 5 cm.