Answer:
3 AU
Step-by-step explanation:
We can solve the problem by using Kepler's third law, which states that the ratio between the cube of the orbital radius and the square of the orbital period is constant for every object orbiting the Sun. So we can write
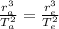
where
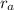 is the distance of the asteroid from the sun (orbital radius)
is the distance of the asteroid from the sun (orbital radius)
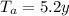 is the orbital period of the asteroid
is the orbital period of the asteroid
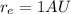 is the orbital radius of the Earth
is the orbital radius of the Earth
 is the orbital period the Earth
is the orbital period the Earth
Solving the equation for
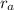 , we find
, we find
![r_a = \sqrt[3]{(r_e^3)/(T_e^2)T_a^2} =\sqrt[3]{((1 AU)^3)/((1 y)^2)(5.2 y)^2}=3 AU](https://img.qammunity.org/2020/formulas/physics/high-school/yzr44sw7ry74fh0bv7gy89l54p1uek1lih.png)
So, the distance of the asteroid from the Sun is exactly 3 times the distance between the Earth and the Sun.