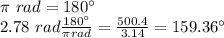Answer:
2.78 radians or 159.36°
Explanation:
Givens:
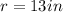
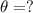
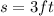
 refers to the length of the circumference, which is the same distance rolled.
refers to the length of the circumference, which is the same distance rolled.
So, to find the angle, we have to use this definition:
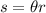 ; which is the relation between arc, angle and length.
; which is the relation between arc, angle and length.
Before, replacing all values, we have to transform 13 inches in feet. We know that 1 feet is 12 inches. So,
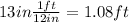
Now, we find the angle.
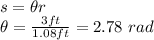
Therefore, the angle is 2.78 radians.
To have it in degrees, we have to transform.