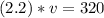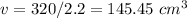Question

The volume of a gas in cubic centimeters v,varies inversely with the pressure of the gas in liters per square centimeters,p when the volume of gas is 16cm3,it’s pressure is 20L/cm2.Find the volume of the gas when the pressure is 2.2L/cm2.

Answer 1

The volume of the gas is
`145.45\ cm^(3)`

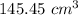
Explanation:

we know that

A relationship between two variables, x, and y, represent an inverse variation if it can be expressed in the form
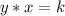`y*x=k` or
`y=k/x`

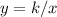
Let

v------> the volume of a gas in cubic centimeters

p-----> the pressure of the gas in liters per square centimeters

so

`p*v=k`

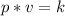
we have

`v=16\ cm^(3), p=20\ l/cm^(2)`

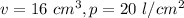
substitute and solve for k

`k=16*20=320`

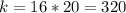
The equation is
`p*v=320`

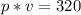
Find the volume of the gas when the pressure is 2.2 L/cm2

so

For
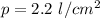`p=2.2\ l/cm^(2)` Find the value of v

substitute in the equation

`(2.2)*v=320`

`v=320/2.2=145.45\ cm^(3)`