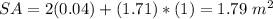Answer:
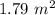
Explanation:
we know that
The surface area of the triangular prism is equal to
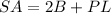
where
B is the area of the triangular base
P is the perimeter of the triangular base
L is the length of the prism
Find the area of the triangular base B
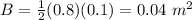
Find the perimeter of the triangular base P
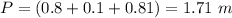
we have
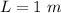
substitute the values and find the surface area