Question

Can someone answer this?

Answer 1

Second option 3; -3

`\lim_(x \to 2^-)f(x)=3`

`\lim_(x \to 2^+)f(x)=-3`

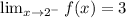
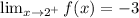
Explanation:

We want to find:

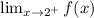`\lim_(x \to 2^+)f(x)` and
`\lim_(x \to 2^-)f(x)`

When x tends to 2 on the left then
`x <2`.

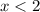When x is less than 2
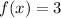`f(x) = 3`. This implies that the limit of f(x) when x approaches 2 from the left is equal to 3. Observe the attached image.

This is:

`\lim_(x \to 2^-)f(x)=3`

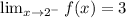
Also When x tends to 2 on the rigtn then
`x >2`.

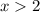When x is greater than 2
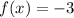`f(x) = -3`. This implies that the limit of f(x) when x approaches 2 from the rigth is equal to -3. This is:

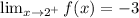`\lim_(x \to 2^+)f(x)=-3` Observe the attached image.