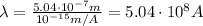Question

As you may well know, placing metal objects inside a microwave oven can generate sparks. Two of your friends are arguing over the cause of the sparking, with one stating that the microwaves \"herd\" electrons into \"pointy\" areas of the metal object, from which the electrons jump from one part of the object to another. The other friend says that the sparks are caused by the photoelectric effect. In this problem, we will prove or disprove the latter idea using basic physics. Suppose the typical work function of the metal is roughly 3.950 × 10-19 J. Calculate the maximum wavelength in angstroms of the radiation that will eject electrons from the metal.

Answer 1

`5.04\cdot 10^8 A`

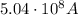
Step-by-step explanation:

The work function of the metal corresponds to the minimum energy needed to extract a photoelectron from the metal. In this case, it is:

`\phi = 3.950\cdot 10^(-19)J`

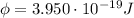
So, the energy of the incoming photon hitting on the metal must be at least equal to this value.

The energy of a photon is given by

`E=(hc)/(\lambda)`

where

h is the Planck's constant

c is the speed of light

`\lambda` is the wavelength of the photon

Using
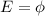`E=\phi` and solving for
`\lambda`, we find the maximum wavelength of the radiation that will eject electrons from the metal:

`\lambda=(hc)/(E)=((6.63\cdot 10^(-34) Js)(3\cdot 10^8 m/s))/(3.950\cdot 10^(-19) J)=5.04\cdot 10^(-7)m`

And since

1 angstrom =
`10^(-15)m`

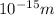
The wavelength in angstroms is

`\lambda=(5.04\cdot 10^(-7) m)/(10^(-15) m/A)=5.04\cdot 10^8 A`