Question

Which statement about y = x2 − x3 is true?

a.It is not a function.

b.It is an even function.

c.It is neither an odd nor an even function.

d.It is an odd function.

Answer 1

Option c. It is neither an odd nor an even function.

Explanation:

The equation
`y = x^2 - x^3` is a function, because there is a single value of y for each value of the domain x.

To test if it is an even function we must do
`y = f(-x)`. If
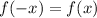`f(-x) = f(x)` then it is an even function.

If
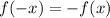`f(-x) = -f(x)` then it is an odd function

`y = f(-x) = (-x) ^ 2 - (- x) ^ 3`

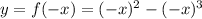
Simplifying we have:

`y = x ^ 2 + x ^ 3`

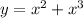
f(-x) is not equal to f(x) so the function is not even.

f(-x) is not equal to -f(x) so the function is not odd.

THE correct answer is the option c

Answer 2

c.It is neither an odd nor an even function.

EXPLANATION

The given function is

`y = f(x) = {x}^(2) - {x}^(3)`

If this function is odd, then f(-a)=-f(a).

`f( - a) = {( - a)}^(2) - {( - a)}^(3)`

`f( - a) = {( a)}^(2) + {( a)}^(3)`

Now ,

`f( a) = {( a)}^(2) - {( a)}^(3)`

`- f( a) = - {( a)}^(2) + {( a)}^(3)`

Since

`f( - a) \\e - f(a)`

The function is not odd.

Also if the function is even, then

`f( a) = f( - a)`

Since

`f( a) \\e f( - a)`

the function is not even.

Hence the function is neither even nor odd.