Question

The height of the triangle is 7 cm longer than its base. the area of the triangle is 60 cm squared. what is the base of the triangle?

Answer 1

Base = 8 cm

Explanation:

We are given that the height of a triangle is 7 cm longer than its base and its area is 60 cm squared.

We are to find the base of the triangle.

Area of the triangle =
`(1)/(2) * base * height`

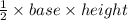
Assuming the base to be
`x`, so height will be
`x+7`

Substituting the values in the above formula to get:

`60=(1)/(2) * x * (x+7)`

`120= x(x+7)`

`x^2+7x-120=0`

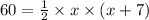
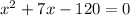
Factorizing the quadratic equation to get:

`x^2+15x-8x-120=0`

`x(x+15)-8(x+15)=0`

`(x-8)(x+15)=0`

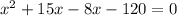
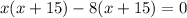
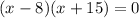
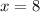`x = 8` and
`x = -15`

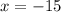
Since base cannot be negative so it will be 8 cm.

Answer 2

8 cm

Explanation:

Let x cm be the length of the base, then the length of the height is x+7 cm. Use formula for the area of the triangle:

`A_(triangle)=(1)/(2)\cdot \text{base}\cdot \text{height}`

In your case,

`60=(1)/(2)\cdot x\cdot (x+7),\\ \\120=x(x+7),\\ \\x^2+7x-120=0,\\ \\D=7^2 -4\cdot 1\cdot (-120)=49+480=529,\\ \\x_(1,2)=(-7\pm√(529))/(2\cdot 1)=(-7\pm 23)/(2)=-15,\ 8`

Since the base cannot have negative length, then x=8 cm.