Question

Is (1, 3) a solution to the system of equations listed below? (1 point) y= 6x -3 y = x - 2

Answer 1

The solution for given system of equation is:
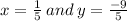`x=(1)/(5)\:and\:y=(-9)/(5)` and ordered pair is:
`\mathbf{((1)/(5),(-9)/(5))}`

So, (1,3) is not solution to the given system of equations.

Explanation:

we can solve the system of equations to find the value of x and y and then verify if (1,3) is a solution or not.

The system of equation given is:

`y=6x-3\\y=x-2`

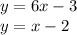
Solving:

Let:

`y=6x-3--eq(1)\\y=x-2--eq(2)`

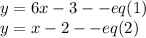
Put value of y from equation 2 into equation 1

`y=6x-3\\Put\:y=x-2\\x-2=6x-3\\x-6x=-3+2\\-5x=-1\\x=(-1)/(-5)\\x=(1)/(5)`

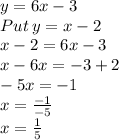
Now, put value of x in equation 2 to find value of y

`y=x-2\\Put\:x=(1)/(5) \\y=(1)/(5) -2\\y=(1-2*5)/(5) \\y=(1-10)/(5)\\ y=(-9)/(5)`

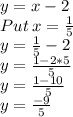
So, the solution for given system of equation is:
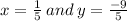`x=(1)/(5)\:and\:y=(-9)/(5)` and ordered pair is:
`\mathbf{((1)/(5),(-9)/(5))}`

So, (1,3) is not solution to the given system of equations.