Question

Using the factor theorem, which of the following is a factor of the polynomial below?

3x^3-4x^2-13x-6

A. X+6
B. X+3
C. X-4
D.X+1

Answer 1

ANSWER

The correct choice is D.

Step-by-step explanation

Let


`f( x) = 3 {x}^(3) - 4 {x}^(2) - 13x - 6`

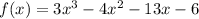
According to the Factor Theorem, if x-a is a factor of f(x), then f(a)=0.

For option A,


`f( - 6) = 3 {( - 6)}^(3) - 4 {( - 6)}^(2) - 13( - 6) - 6`


`f( - 6) = - 720`

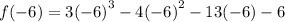
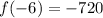
Hence x+6 is not a factor.

For option B,


`f( - 3) = 3 {( - 3)}^(3) - 4 {( - 3)}^(2) - 13( - 3) - 6`


`f( - 3) = - 84`

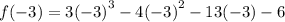
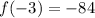
x+3 is also not a factor.

For option C,


`f( 4) = 3 {( 4)}^(3) - 4 {( 4)}^(2) - 13( 4) - 6`


`f(4) = 70`

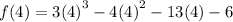
Option D,


`f( - 1) = 3 {( - 1)}^(3) - 4 {( - 1)}^(2) - 13( - 1) - 6`


`f( - 1) = - 3 - 4 + 13 - 6`


`f( - 1) = 0`

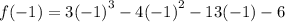
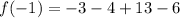
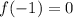
x+1 is a factor.