Question

Given: arc IV =140°, arc VK =30°, m∠ISV=135, Find: m∠VPL

Answer 1

The measure of the
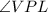`{\angle}VPL` is
`80^(\circ)`

Explanation:

We know that the measure of the inner angle is the semi-sum of the arcs comprising it and its opposite.

Now, Let

x is the measure of arc IV

and y is the measure of arc PK, then

`m{\angle}ISV={(1)/(2)}(x+y)`

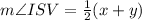
Substituting the given values, we get

`135^(\circ)={(1)/(2)(140^(\circ)+y)`

`270^(\circ)=140^(\circ)+y`

`y=130^(\circ)`

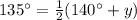
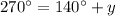
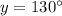
Thus, The measure of arc PK is
`130^(\circ)`.

Also, we know that the inscribed angle measures half that of the arc comprising , thus

Let

z is the measure of arc VK

and y is the measure of arc PK, then

`m{\angle}VPL={(1)/(2)(z+y)`

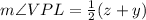
Substituting the values, we get

`m{\angle}VPL={(1)/(2)}(30+130)=80^(\circ)`

Hence, the measure of the
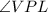`{\angle}VPL` is
`80^(\circ)`.