For this case we must factor the following expression:
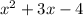
To factor, we must find two numbers that when added together result in +3. And when multiplied, give -4 as a result.
Those numbers are +4 and -1, then:
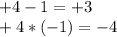
Thus, the factorization remains:
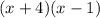
We check applying distributive property, then:
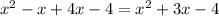
Answer:
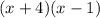
Option C